Young's Modulus: Definition, Values, and Examples

Young's modulus is a mathematical/engineering definition for one of the simplest methods of understanding elasticity. Elasticity is the ability of a material to undergo a change of shape when a force is applied to it and revert to its original shape/dimensions when the force is removed. Note that all values of Young’s modulus are derived from the elastic range of the materials’ loaded behavior. Some materials have no elastic range, others move from elastic to plastic or brittle behavior very quickly, whereas others have a clearly defined elastic range before an internal process of atomic displacement begins a plastic phase. Examples of Young’s modulus values (in gigapascals, GPa) are: ABS (1.4–3.1 GPa), aluminum (69 GPa), and nylon (2–4 GPa).

Specifically, Young’s modulus is a measure of the elasticity of wires/strands. It is hard to compare or even develop values for larger or more complex cross-sections. But, it is an excellent tool for relative analysis of simple material samples—allowing close analysis of the effects of processing methods, alloying agents, and heat treatments for example. This article will discover, Just what is Young’s modulus? It’ll also define how the modulus is calculated, and provide some examples.

What Is Young's Modulus?

Young's modulus is a measure of the force per unit extension, taking into account cross-sectional area. This measurement is a systematic way to define the elastic behavior of any material, allowing comparisons between disparate material types/families.

What Is the SI Unit of Young's Modulus?

In SI form, Young’s modulus is measured using the pascal (Pa), or more usually the gigapascal (GPa). It is also the standard unit of pressure.

What Is an Example of Young's Modulus?

A classic example of the use of Young’s modulus is in calculating the safe working limits of cables used in cranes, cable cars, and ski lifts. A good understanding of the effect of loading on such cables is important to operate them safely. Ensuring that the safe loads never exceed the elastic limit capacity of the cables is a critical design/operational parameter, usually with an FoS (Factor of Safety) which is defined by strict risk assessments and can be between 1.5 and 10. Figure 1 shows an example of Young’s modulus:

What Are the Young's Modulus Values?

Young's modulus values cover a huge range, but they still provide a well-used measure of the elastic capability of many materials. The lowest values of Young’s modulus are for materials like natural rubber, at 0.01–0.1 GPa, whereas the highest values are typically for carbon nanotube materials (up to 1,000 GPa). Other examples are for obscure metals (such as iridium, 570 GPa) and for carefully alloyed and heat-treated steels used in spring manufacture (up to 220 GPa).

How Does the Values of Young's Modulus Expressed?

Young’s modulus is expressed as pressure. In the metric system, this is in pascals or gigapascals (Pa or GPa). In American/imperial units, pressure is expressed in pounds per square inch (PSI)

What Material Has the Highest Young's Modulus?

Diamond is considered to have the highest Young’s modulus at around 1,210 GPa. A material identified in several meteorites is a carbon allotrope like a diamond, but instead of being cubic in structure, it is formed from a hexagonal carbon matrix. This material, named Lonsdaleite, doesn’t yet exist in samples large enough to be Young’s modulus tested, but it is known to be harder and stronger than diamond.

What Is a Large Young's Modulus Value Indicates?

A high Young's modulus value indicates the high stiffness of a material and its resistance to (elastic) deformation under load. A high value of Young’s modulus points to a material that does not stretch easily.

What Is a Small Young's Modulus Value Indicates?

A low Young's modulus value indicates a material that undergoes large (elastic) deformation under a relatively low load. Such materials stretch easily. Natural rubbers stretch very easily, confirmed by a low Young’s modulus value. Some silicone rubbers have almost unmeasurably low Young’s modulus values, stretching under their own weight.

What Is the Young's Modulus Symbol?

Young’s modulus is expressed as a capital E (Epsilon) or, less commonly, Y (Young).

How Does the Young's Modulus Determined?

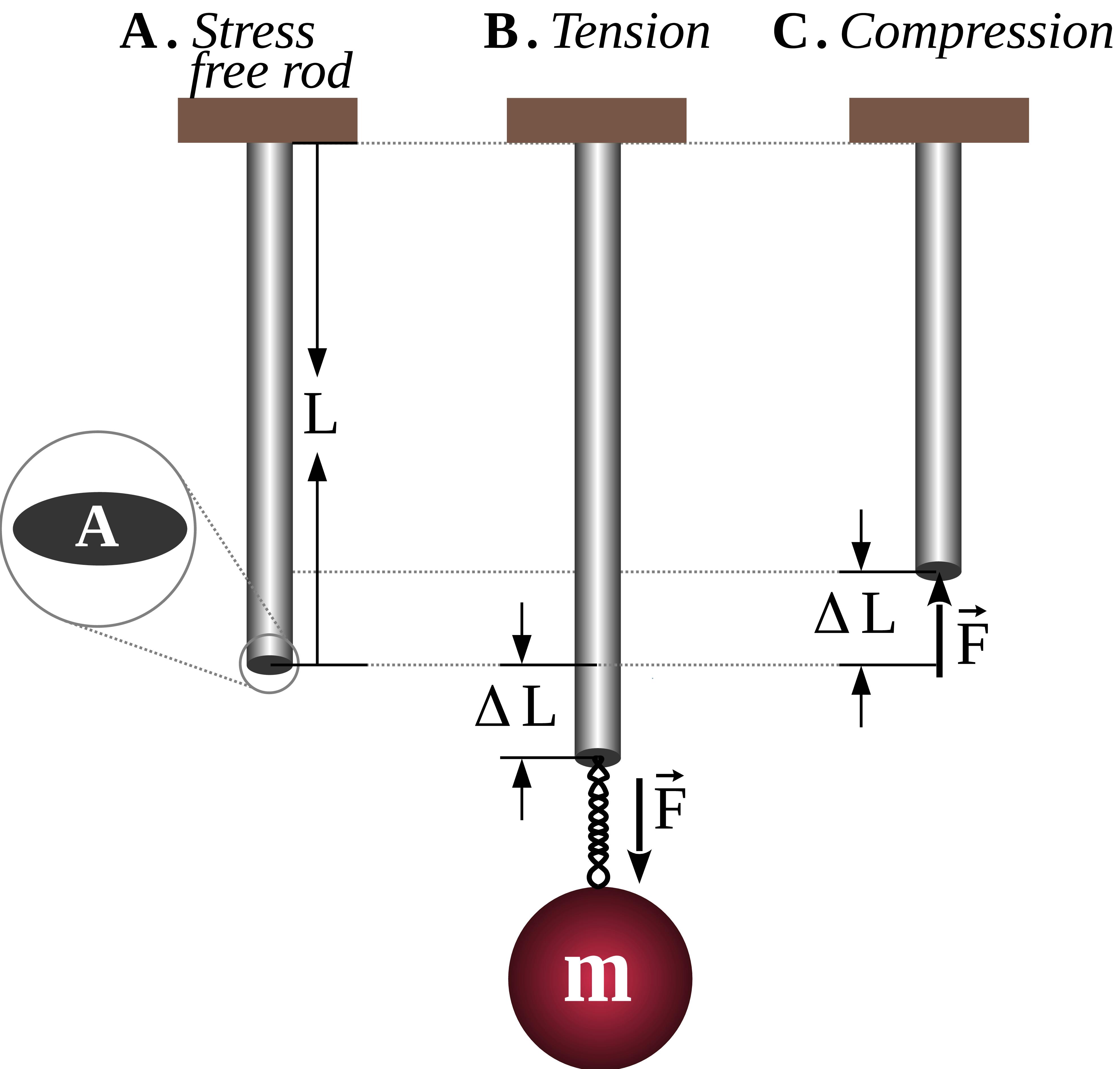
Young’s modulus is determined by suspending a wire/thread/strand of the uniform cross-sectional area from a strong point and loading the lower end with enough weight to just straighten it. Weights are then added and measurements of extension are taken. It is important to validate that the elastic limit of the material is not exceeded, or the numbers will include some plastic deformation and will be invalid as an informative test.

From the values of load, extension, and cross-sectional area, two values can be calculated:

• Stress, σ, is defined as the force per unit area. This is calculated by applying two load values to tension the strand under test and dividing the Δ load (the load increase) by the cross-sectional area of the strand:

With dimensions in pascals or PSI

• Strain, ϵ, is defined as the extension per unit length. Measuring the total length of the strand at the start load and end load gives a difference in length:

What Is the Young's Modulus Equation?

E or Y, Young’s modulus for material is calculated from the below equation:

Where: 

σ = stress

ϵ = strain

What Is the Difference Between Young's Modulus and Shears Modulus?

The shear modulus of elasticity is a similar measure to Young’s modulus. But, in place of the pure tensile loading used to evaluate Young’s modulus, a sample is loaded in pure shear. This can, for example, be achieved by applying a pure torsional load to a bar or tube. And shear modulus testing is performed on a wider spectrum of cross-sections than Young’s modulus testing.

Many materials have spring properties (i.e., the capacity to undergo purely elastic deformation that entirely recovers when loading is removed). It is clear that materials that can operate as linear springs can also operate as springs in a torsional loading mode.

The shear modulus of elasticity can be just as informative as Young’s modulus when investigating the elastic behaviors of materials. The selection of materials for torsional loading scenarios is just as important as for tensile loads. In fact, loading in real systems is rarely uni-axial, so a deeper understanding of material behaviors can result in much better-informed design decisions and material selections.

Summary

This article presented Young's modulus, explained what it is, and discussed how to measure it on various materials. To learn more about Young's modulus, contact a Xometry representative.

Xometry provides a wide range of manufacturing capabilities, including 3D printing and other value-added services for all of your prototyping and production needs. Visit our website to learn more or to request a free, no-obligation quote.

Disclaimer

The content appearing on this webpage is for informational purposes only. Xometry makes no representation or warranty of any kind, be it expressed or implied, as to the accuracy, completeness, or validity of the information. Any performance parameters, geometric tolerances, specific design features, quality and types of materials, or processes should not be inferred to represent what will be delivered by third-party suppliers or manufacturers through Xometry’s network. Buyers seeking quotes for parts are responsible for defining the specific requirements for those parts. Please refer to our terms and conditions for more information.